Discrete Random Variables And Probability Distributions

Probability Distribution Of Discrete Random Variables Pdf Random variable is said to be discrete if its set of possible values is a discrete set. possible value means a value x0 so that p(x = x0) , 0. we will often write p(x) instead of px(x). note. 3.2 probability distribution of a discrete random variable every discrete random variable, y, a probabil ity mass function (or probability distribution) that gives the probability that yis exactly equal to some value. (def 3.2 and 3.3) the probability that a dis crete y takes on the value y, p(y) = p(y = y),.

Discrete Random Variables Pdf Probability Distribution Random The probability distribution of a discrete random variable \(x\) is a list of each possible value of \(x\) together with the probability that \(x\) takes that value in one trial of the experiment. the probabilities in the probability distribution of a random variable \(x\) must satisfy the following two conditions:. Two types of random variables •a discrete random variable has a countable number of possible values •a continuous random variable takes all values in an interval of numbers. In this chapter, we discussed basic concepts and properties of probability distributions for discrete random variables. important discrete distributions such as the binomial, hypergeometric, and poisson distributions are discussed in detail. applications of these distributions in business decisions are also examined. A probability distribution describes how probabilities are distributed across the different values or outcomes of a (random) variable. it shows how likely the different values or outcomes are, highlighting the most and least probable outcomes. since a random variable can be discrete (e.g. the number of children in a family) or continuous (e.g.
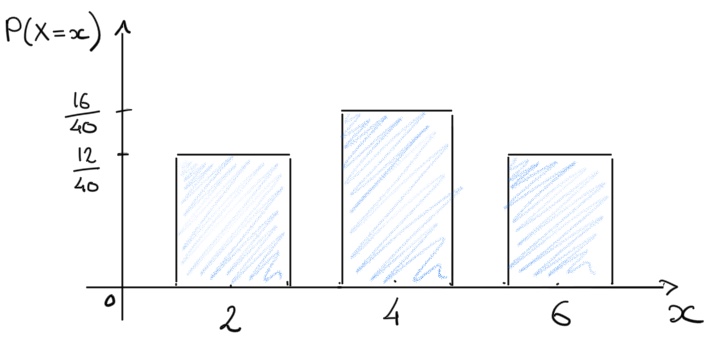
Discrete Random Variables Probability Distribution Functions In this chapter, we discussed basic concepts and properties of probability distributions for discrete random variables. important discrete distributions such as the binomial, hypergeometric, and poisson distributions are discussed in detail. applications of these distributions in business decisions are also examined. A probability distribution describes how probabilities are distributed across the different values or outcomes of a (random) variable. it shows how likely the different values or outcomes are, highlighting the most and least probable outcomes. since a random variable can be discrete (e.g. the number of children in a family) or continuous (e.g. Random variable: a random variable x is a real valued function on the sample space s. that is, x : s ! r; where r is the set of all real numbers. note that the value of a random variable depends on a random event. a rv x is discrete if we can list its all possible values; that is, it assumes only distinct (finite or countable) values. Probability distributions for discrete random variables probabilities assigned to various outcomes in the sample space s, in turn, determine probabilities associated with the values of any particular random variable defined on s. the probability mass function (pmf) of x , p(x) describes how the total probability is distributed among all the. 1. discrete random variable a random variable (r.v.) is a real valued function defined over a sample space. ar.v.y is said to be discrete if it can assume only a finite or countably infinite number of distinct values. 2. probability distribution (p.d.) for a discrete r.v. y can be represented by a formula, a table, or a graph which provides the. Overview of probability distributions. to begin our discussion of probability distributions, some terminology will be helpful: random variable —a variable where a single numerical value is assigned to a specific outcome from an experiment. typically the letter x x is used to denote a random variable. for example, assign the numerical values 1, 2, 3, … 13 to the cards selected from a.

02 Discrete Probability Distribution Pdf Probability Distribution Random variable: a random variable x is a real valued function on the sample space s. that is, x : s ! r; where r is the set of all real numbers. note that the value of a random variable depends on a random event. a rv x is discrete if we can list its all possible values; that is, it assumes only distinct (finite or countable) values. Probability distributions for discrete random variables probabilities assigned to various outcomes in the sample space s, in turn, determine probabilities associated with the values of any particular random variable defined on s. the probability mass function (pmf) of x , p(x) describes how the total probability is distributed among all the. 1. discrete random variable a random variable (r.v.) is a real valued function defined over a sample space. ar.v.y is said to be discrete if it can assume only a finite or countably infinite number of distinct values. 2. probability distribution (p.d.) for a discrete r.v. y can be represented by a formula, a table, or a graph which provides the. Overview of probability distributions. to begin our discussion of probability distributions, some terminology will be helpful: random variable —a variable where a single numerical value is assigned to a specific outcome from an experiment. typically the letter x x is used to denote a random variable. for example, assign the numerical values 1, 2, 3, … 13 to the cards selected from a.

Discrete Probability Distribution Chapter3 Pdf Probability 1. discrete random variable a random variable (r.v.) is a real valued function defined over a sample space. ar.v.y is said to be discrete if it can assume only a finite or countably infinite number of distinct values. 2. probability distribution (p.d.) for a discrete r.v. y can be represented by a formula, a table, or a graph which provides the. Overview of probability distributions. to begin our discussion of probability distributions, some terminology will be helpful: random variable —a variable where a single numerical value is assigned to a specific outcome from an experiment. typically the letter x x is used to denote a random variable. for example, assign the numerical values 1, 2, 3, … 13 to the cards selected from a.

Chapter 3 Discrete Probability Distribution Pdf Probability

Comments are closed.