Draw A Triangle And The Perpendicular Bisectors Of All Three Sides Do

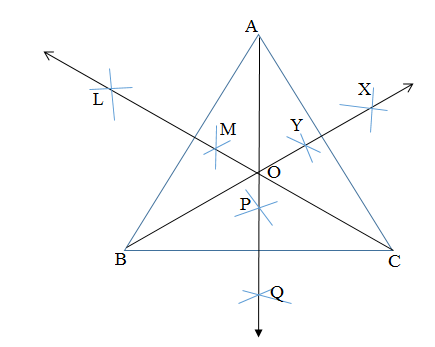
Draw A Triangle And The Perpendicular Bisectors Of All Three Sides Do Perpendicular bisectors of sides of a triangle meet at a point. To do the perpendicular bisector of a triangle (acute, right or obtuse triangle) we need a ruler and a compass. steps. place the ruler where the two arcs cross and draw the line segment s joining the points p and q. this is the perpendicular bisector of the side ab.

Draw A Triangle And Perpendicular Bisectors Of All Three Sides Do All The perpendicular of a triangle is perpendicular to the sides drawn from the opposite vertices and divides the sides into two equal parts. the point at which all the three perpendicular bisectors of a triangle meets is called the circumcenter of a triangle . Investigation 5 1: constructing the perpendicular bisectors of the sides of a triangle. tools needed: paper, pencil, compass, ruler. 1. draw a scalene triangle. 2. construct the perpendicular bisector (investigation 1 3) for all three sides. the three perpendicular bisectors all intersect at the same point, called the circumcenter. Draw a triangle and the perpendicular bisector all three sides , do all three bisectors intersect at the same point? step by step explanation: the three perpendicular bisectors all intersect at the same point, called the circumcenter. circumcenter: the point of concurrency for the perpendicular bisectors of the sides of a triangle. Construct the perpendicular bisector (investigation 1 3) for all three sides. the three perpendicular bisectors all intersect at the same point, called the circumcenter. circumcenter: the point of concurrency for the perpendicular bisectors of the sides of a triangle.

A Draw An Acute Triangle Construct The Perpendicular Bisec Quizlet Draw a triangle and the perpendicular bisector all three sides , do all three bisectors intersect at the same point? step by step explanation: the three perpendicular bisectors all intersect at the same point, called the circumcenter. circumcenter: the point of concurrency for the perpendicular bisectors of the sides of a triangle. Construct the perpendicular bisector (investigation 1 3) for all three sides. the three perpendicular bisectors all intersect at the same point, called the circumcenter. circumcenter: the point of concurrency for the perpendicular bisectors of the sides of a triangle. Construct the perpendicular bisectors of all three sides of abc. then drag the vertices to change abc. what do you notice about the perpendicular bisectors? b. label a point d at the intersection of the perpendicular bisectors. c. draw the circle with center d through vertex a of abc. then drag the vertices to change abc. what do you notice?. Investigation 5 1: constructing the perpendicular bisectors of the sides of a triangle. tools needed: paper, pencil, compass, ruler 1. draw a scalene triangle. 2. construct the perpendicular bisector (investigation 1 3) for all three sides. the three perpendicular bisectors all intersect at the same point, called the circumcenter. Properties of the perpendicular bisectors of a triangle. work with a partner: use dynamic geometry software. draw any ∆abc. a. construct the perpendicular bisectors of all three sides of ∆abc. then drag the vertices to change ∆abc. ‘what do you notice about the perpendicular bisectors? answer: b. Draw the perpendicular bisectors of line segments 𝐴𝐵, 𝐵𝐶, and 𝐴𝐶. what do you observe about the three perpendicular bisectors? (a) they all intersect at one point. (b) they are all equal in length. (c) they are all medians of the triangle.

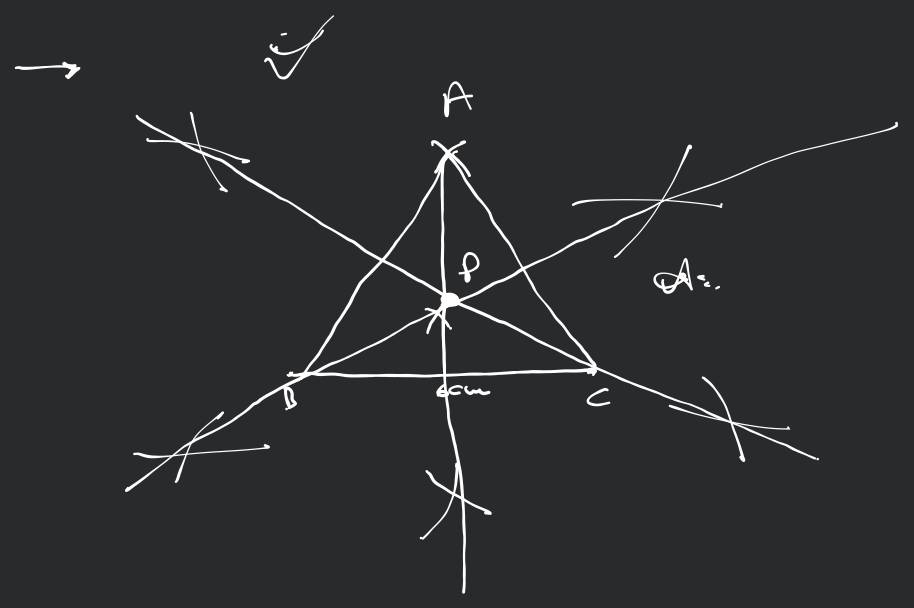
Draw A Triangle Construct Three Perpendicular Bisectors On Each Of Its S Construct the perpendicular bisectors of all three sides of abc. then drag the vertices to change abc. what do you notice about the perpendicular bisectors? b. label a point d at the intersection of the perpendicular bisectors. c. draw the circle with center d through vertex a of abc. then drag the vertices to change abc. what do you notice?. Investigation 5 1: constructing the perpendicular bisectors of the sides of a triangle. tools needed: paper, pencil, compass, ruler 1. draw a scalene triangle. 2. construct the perpendicular bisector (investigation 1 3) for all three sides. the three perpendicular bisectors all intersect at the same point, called the circumcenter. Properties of the perpendicular bisectors of a triangle. work with a partner: use dynamic geometry software. draw any ∆abc. a. construct the perpendicular bisectors of all three sides of ∆abc. then drag the vertices to change ∆abc. ‘what do you notice about the perpendicular bisectors? answer: b. Draw the perpendicular bisectors of line segments 𝐴𝐵, 𝐵𝐶, and 𝐴𝐶. what do you observe about the three perpendicular bisectors? (a) they all intersect at one point. (b) they are all equal in length. (c) they are all medians of the triangle.