How To Find Normal Vector Of A Surface
Surface Vector At Vectorified Collection Of Surface Vector Free “a normal vector is a vector that is perpendicular to another surface, vector, or axis, in short, making an angle of 90° with the surface, vector, or axis.” in this section of normal vectors, we will be covering the following topics: what is a normal vector? how to find a normal vector? what is the formula of normal vectors? examples. Learn what a normal vector is and how to find it for different types of surfaces, such as planes, curves and parametric surfaces. see the formulas, equations and references for calculating normal vectors and unit normals.
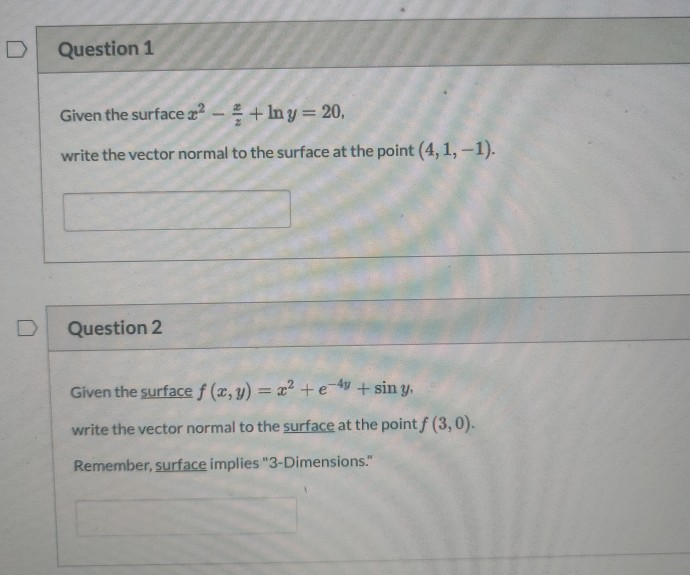
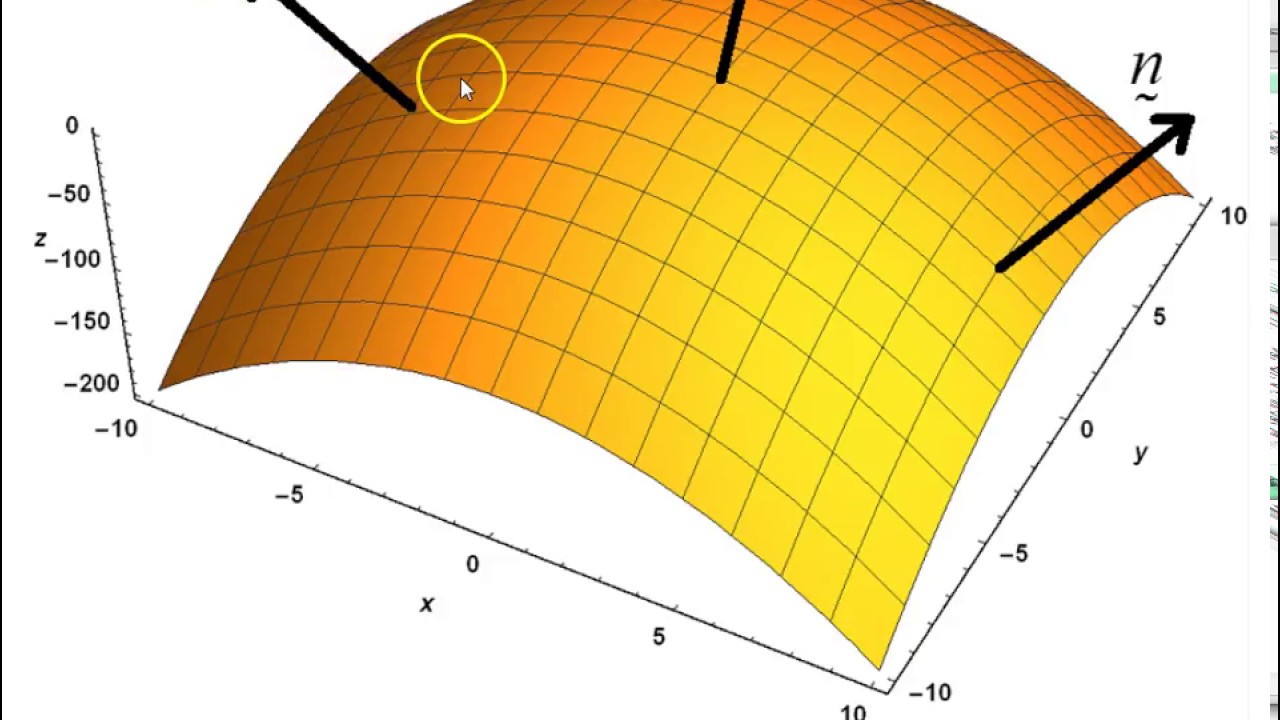
Solved 1 Find The Vector Normal To The Surface At Point Chegg Finding the normal to a surface one of the elements of solving surface integrals in vector calculus is determining the normal to a surface so that we can evaluate the flux of a vector through that surface. we can write our surface as some function : f = where c is a constant. for example, the equation of a plane has. The easiest surface is probably the top part of the cylinder, lying above the sphere; the normal to the cylinder is easy. but you can choose any surface, such as the portion of the sphere enclosed by your curve. In three dimensional space, a surface normal, or simply normal, to a surface at point p is a vector perpendicular to the tangent plane of the surface at p. the vector field of normal directions to a surface is known as gauss map. Suppose our surface involved 1 variable, instead of 3, such as f = x2 a2 f = x 2 a 2. how would we find the normal vector at a given point? what we would want to do, is find the tangent line, and then find the vector perpendicular to it. now what does the tangent line look like?.

Solved 30 35 Curve And Surface Normal Vectors Find A Normal Chegg In three dimensional space, a surface normal, or simply normal, to a surface at point p is a vector perpendicular to the tangent plane of the surface at p. the vector field of normal directions to a surface is known as gauss map. Suppose our surface involved 1 variable, instead of 3, such as f = x2 a2 f = x 2 a 2. how would we find the normal vector at a given point? what we would want to do, is find the tangent line, and then find the vector perpendicular to it. now what does the tangent line look like?. In this section, we explore the concept of a normal vector to a surface and its use in nding equations of tangent planes. to begin with, a level surface u (x; y; z) = k is said to be smooth if the gradient ru = hux; is continuous and non zero at any point on the surface. It begins with calculating the usual normal vector in euclidean space using vector calculus before going on to explain the equivalent calculation in curved spaces using the covariant derivative . Courses on khan academy are always 100% free. start practicing—and saving your progress—now: khanacademy.org math multivariable calculus integra.

Comments are closed.