So What Are Complex Numbers

Complex Numbers In mathematics, a complex number is an element of a number system that extends the real numbers with a specific element denoted i, called the imaginary unit and satisfying the equation =; every complex number can be expressed in the form , where a and b are real numbers. Complex numbers are the numbers that are expressed in the form of a ib where, a,b are real numbers and ‘i’ is an imaginary number called “iota”. the value of i = (√ 1). for example, 2 3i is a complex number, where 2 is a real number (re) and 3i is an imaginary number (im).
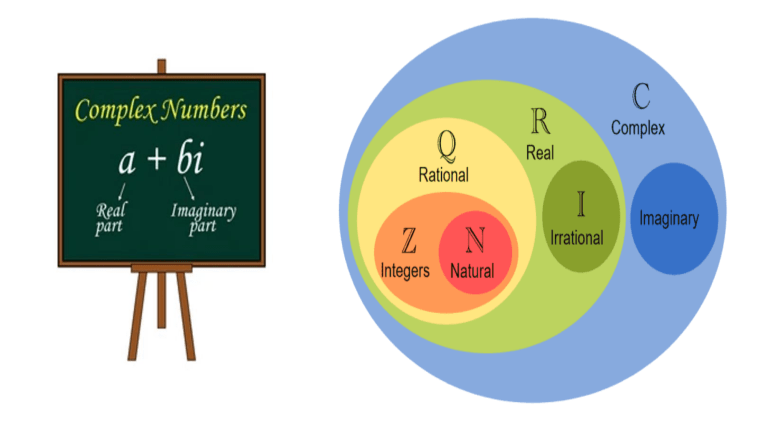
Complex Numbers Diagram Real Imaginary Parts When we combine a real number and an imaginary number we get a complex number: can a number be a combination of two numbers? can we make a number from two other numbers? sure we can! we do it with fractions all the time. the fraction 3 8 is a number made up of a 3 and an 8. we know it means "3 of 8 equal parts". What is a complex number? complex numbers have both a real part and an imaginary part. for example: the real part is 3 and the imaginary part is 4. note that the imaginary part does not include the ' ' complex numbers are often denoted by and we can refer to the real and imaginary parts respectively using and in general:. The complex numbers are the field c of numbers of the form x iy, where x and y are real numbers and i is the imaginary unit equal to the square root of 1, sqrt( 1). when a single letter z=x iy is used to denote a complex number, it is sometimes called an "affix." in component notation, z=x iy can be written (x,y). Complex numbers are an essential concept in mathematics, extending the idea of numbers to include solutions for equations that don’t have real solutions. complex numbers have applications in many scientific research areas, signal processing, electromagnetism, fluid dynamics, quantum mechanics, and vibration analysis.

What Are Complex Numbers The complex numbers are the field c of numbers of the form x iy, where x and y are real numbers and i is the imaginary unit equal to the square root of 1, sqrt( 1). when a single letter z=x iy is used to denote a complex number, it is sometimes called an "affix." in component notation, z=x iy can be written (x,y). Complex numbers are an essential concept in mathematics, extending the idea of numbers to include solutions for equations that don’t have real solutions. complex numbers have applications in many scientific research areas, signal processing, electromagnetism, fluid dynamics, quantum mechanics, and vibration analysis. In introducing complex numbers, and the notation for them, this article brings together into one bigger 'picture' some closely related elementary ideas like vectors and the exponential and trigonometric functions and their derivatives. Complex numbers are numbers that consist of two parts — a real number and an imaginary number. complex numbers are the building blocks of more intricate math, such as. So in order to take square roots of negative numbers, mathematicians have introduced something called complex numbers. complex numbers are based on the imaginary unit i. the imaginary unit i is defined as i 2 = − 1. imaginary numbers are multiples of the imaginary unit. every imaginary number can be written as. where a ∈ ℝ. Complex numbers can be thought of as expressions that contain both real and imaginary parts, typically written as the expression \(a bi\) , where i represents the imaginary unit satisfying \(i^2 = 1\). complex numbers were first invented to solve equations that cannot be solved using real numbers alone, such as solving equations like \(x^2 1.
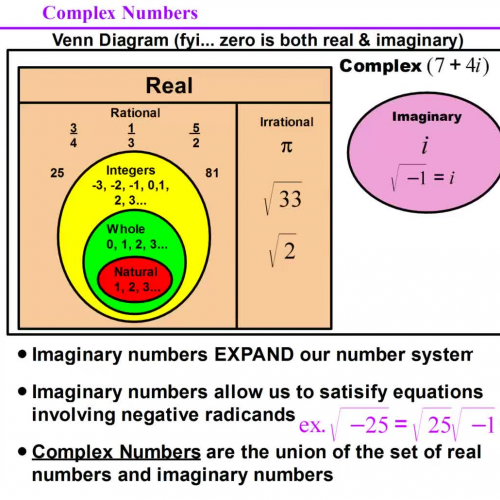
Complex Numbers In introducing complex numbers, and the notation for them, this article brings together into one bigger 'picture' some closely related elementary ideas like vectors and the exponential and trigonometric functions and their derivatives. Complex numbers are numbers that consist of two parts — a real number and an imaginary number. complex numbers are the building blocks of more intricate math, such as. So in order to take square roots of negative numbers, mathematicians have introduced something called complex numbers. complex numbers are based on the imaginary unit i. the imaginary unit i is defined as i 2 = − 1. imaginary numbers are multiples of the imaginary unit. every imaginary number can be written as. where a ∈ ℝ. Complex numbers can be thought of as expressions that contain both real and imaginary parts, typically written as the expression \(a bi\) , where i represents the imaginary unit satisfying \(i^2 = 1\). complex numbers were first invented to solve equations that cannot be solved using real numbers alone, such as solving equations like \(x^2 1.
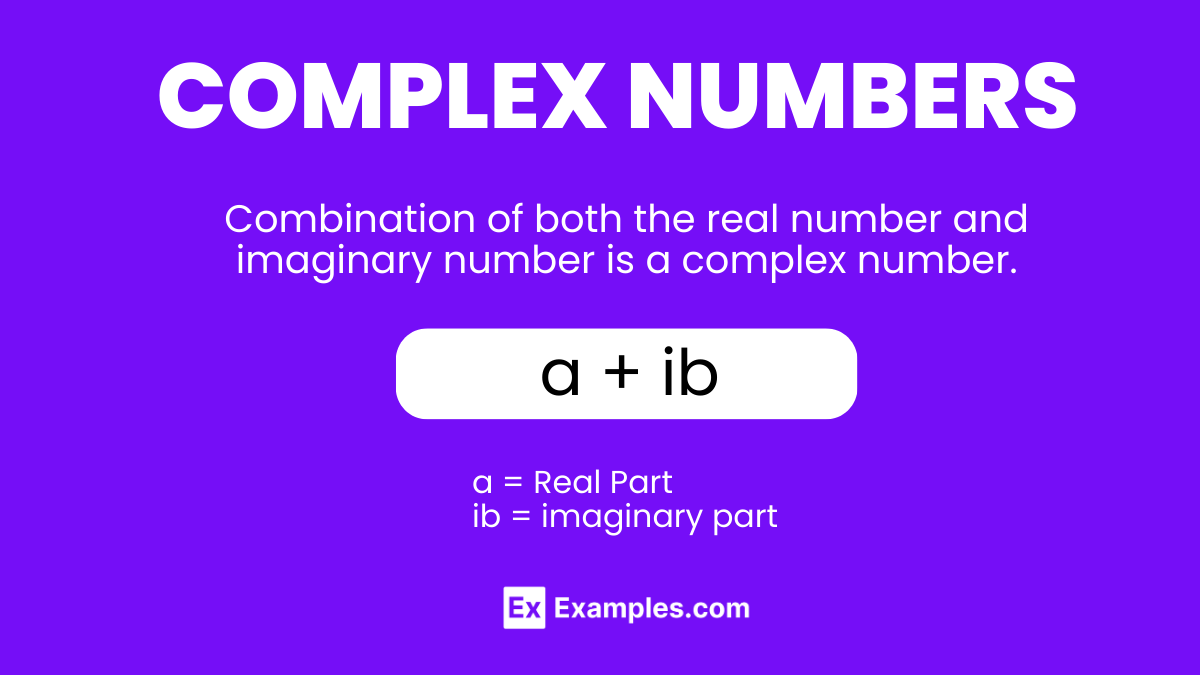
Complex Numbers Formula Notation Differences Graphical Representation So in order to take square roots of negative numbers, mathematicians have introduced something called complex numbers. complex numbers are based on the imaginary unit i. the imaginary unit i is defined as i 2 = − 1. imaginary numbers are multiples of the imaginary unit. every imaginary number can be written as. where a ∈ ℝ. Complex numbers can be thought of as expressions that contain both real and imaginary parts, typically written as the expression \(a bi\) , where i represents the imaginary unit satisfying \(i^2 = 1\). complex numbers were first invented to solve equations that cannot be solved using real numbers alone, such as solving equations like \(x^2 1.

Comments are closed.