Standard Deviation Formula Statistics Variance Sample And Population Standard deviation in statistics, typically denoted by σ, is a measure of variation or dispersion (refers to a distribution's extent of stretching or squeezing) between values in a set of data. the lower the standard deviation, the closer the data points tend to be to the mean (or expected value), μ. Standard deviation formulas for populations and samples. different formulas are used for calculating standard deviations depending on whether you have collected data from a whole population or a sample. population standard deviation.
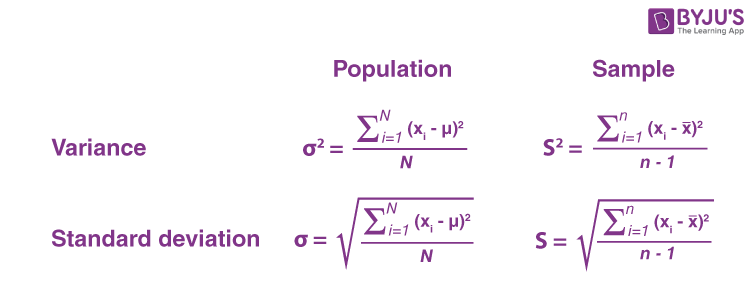
Standard Deviation Formula Statistics Variance Sample Vrogue Co The formula for the (sample) standard deviation (sd) is s = s p n i=1 (x i −x)2 n−1 why divide by n−1? not ? • short answer: one cannot measure variability with only one observation (n = 1). we need at least 2. • long answer: dividing by n would underestimate the true (population) standard deviation. dividing by n−1 instead of. Here we will learn about standard deviation, population, and sample, their various formulas, steps to calculate them, and much more. what is variance? in mathematical terms, the standard deviation is the square root of the variance. variance is the average of the squared differences from the mean. To understand the meaning of the formulas for the mean and standard deviation of the sample mean. suppose we wish to estimate the mean μ of a population. in actual practice we would typically take just one sample. imagine however that we take sample after sample, all of the same size n, and compute the sample mean ˉx each time. For a set of data, the measure of dispersion, about mean, when expressed as the positive square root of the variance, is called standard deviation. it measures the dispersion or spread of data. the formula for calculating the standard deviation of given data is: standard deviation formula. the population standard deviation formula is given as:.
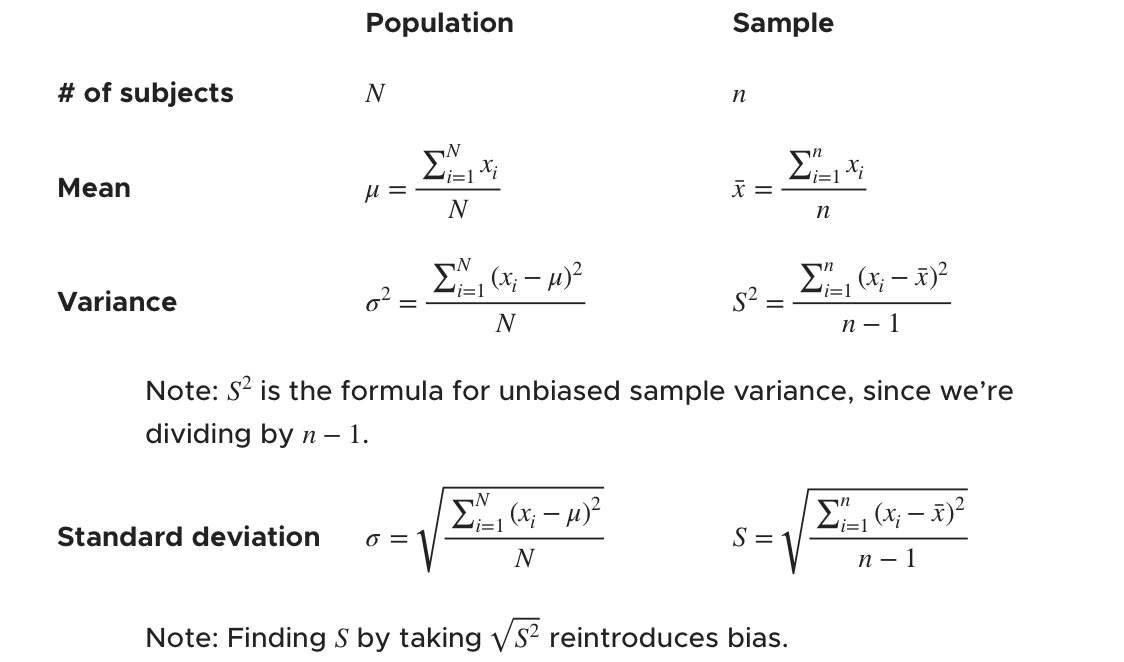
Standard Deviation Formula Statistics Variance Sample Vrogue Co To understand the meaning of the formulas for the mean and standard deviation of the sample mean. suppose we wish to estimate the mean μ of a population. in actual practice we would typically take just one sample. imagine however that we take sample after sample, all of the same size n, and compute the sample mean ˉx each time. For a set of data, the measure of dispersion, about mean, when expressed as the positive square root of the variance, is called standard deviation. it measures the dispersion or spread of data. the formula for calculating the standard deviation of given data is: standard deviation formula. the population standard deviation formula is given as:. Deviation means how far from the normal. standard deviation. the standard deviation is a measure of how spread out numbers are. its symbol is σ (the greek letter sigma) the formula is easy: it is the square root of the variance. so now you ask, "what is the variance?" variance. the variance is defined as:. While finding the standard deviation of a data set, we may encounter two different sets of data based on its scope and size. the two types are population standard deviation and sample standard deviation. let us now discuss them in detail. To calculate the standard deviation of those numbers: 1. work out the mean (the simple average of the numbers) 2. then for each number: subtract the mean and square the result. 3. then work out the mean of those squared differences. 4. take the square root of that and we are done! the formula actually says all of that, and i will show you how. 🧮 the standard deviation formula (simplified) here’s the basic formula for a sample standard deviation: sd = √[ Σ(x x̄)² (n 1) ] let’s translate that into real people terms: x = each number in your data; x̄ (x bar) = the mean (average) n = how many numbers you have; Σ = the fancy symbol for “add up all of this” in plain.
Comments are closed.