Stats How To Find Variance
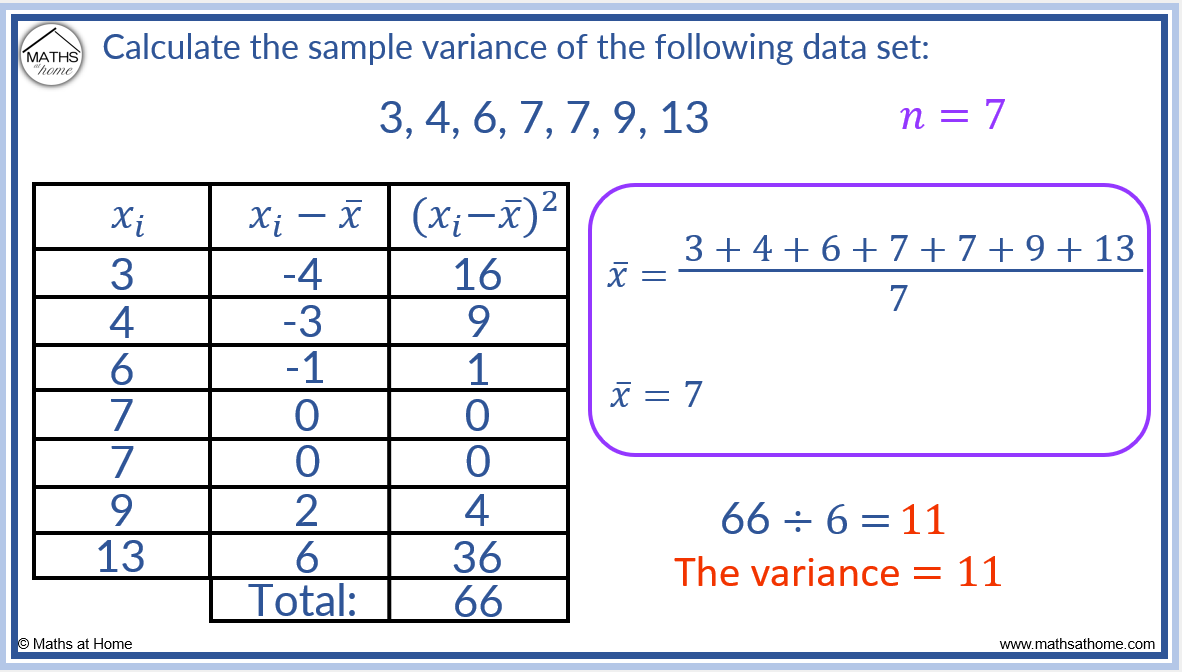
How To Calculate Variance Mathsathome There are five main steps for finding the variance by hand. we’ll use a small data set of 6 scores to walk through the steps. to find the mean, add up all the scores, then divide them by the number of scores. subtract the mean from each score to get the deviations from the mean. since x̅ = 50, take away 50 from each score. Variance is a measure of how spread out a data set is, and we calculate it by finding the average of each data point's squared difference from the mean. it's useful when creating statistical models since low variance can.
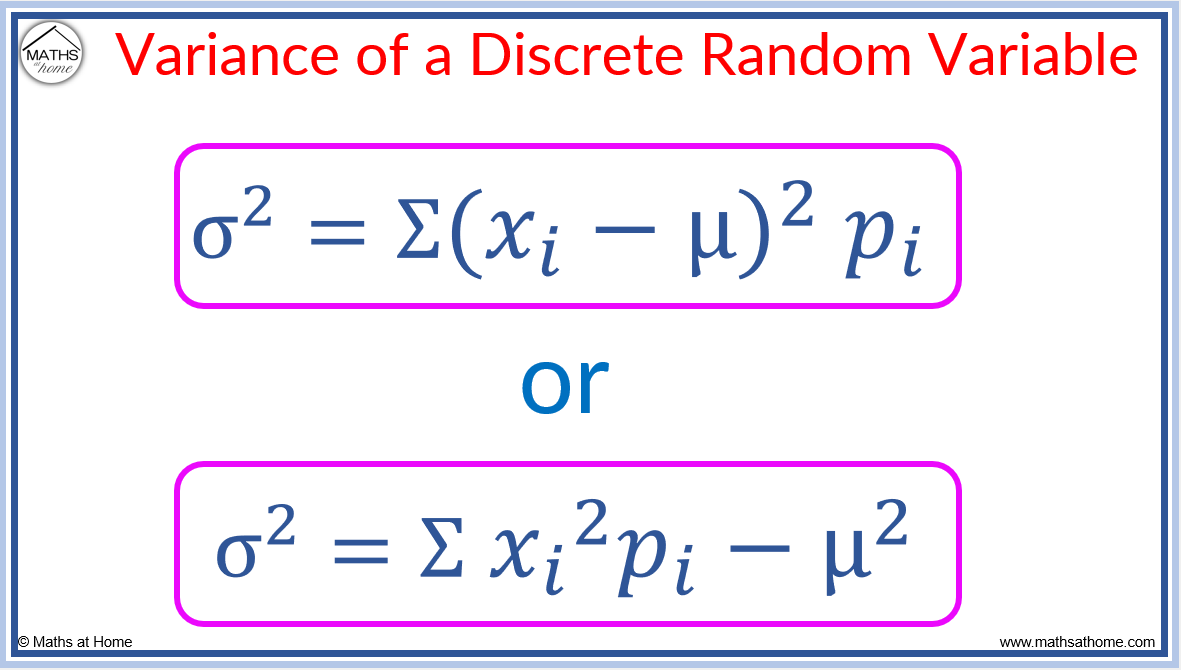
How To Calculate Variance Mathsathome Variance is the sum of squares divided by the number of data points. the formula for variance of a is the sum of the squared differences between each data point and the mean, divided by the number of data values. this calculator uses the formulas below in its variance calculations. for a complete population divide by the size n. What is variance? variance tells us how spread out the numbers are in a data set. if your numbers are all close to the average (mean), you’ll have a low variance. if the numbers are all over the place, your variance will be higher. it’s used in statistics, science, finance, sports, and pretty much any field that works with data!. Variance measures how far a data set is spread out. definition, examples of variance. step by step examples and videos; statistics made simple!. When we want to find how each data point in a given population varies or is spread out, then we use the population variance. it is used to give the squared distance of each data point from the population mean. the formula for population variance is written as, \sigma^2 = \dfrac {\sum (x i – \bar {x})^2 } {n} σ2 = n∑(xi–xˉ)2. where,.
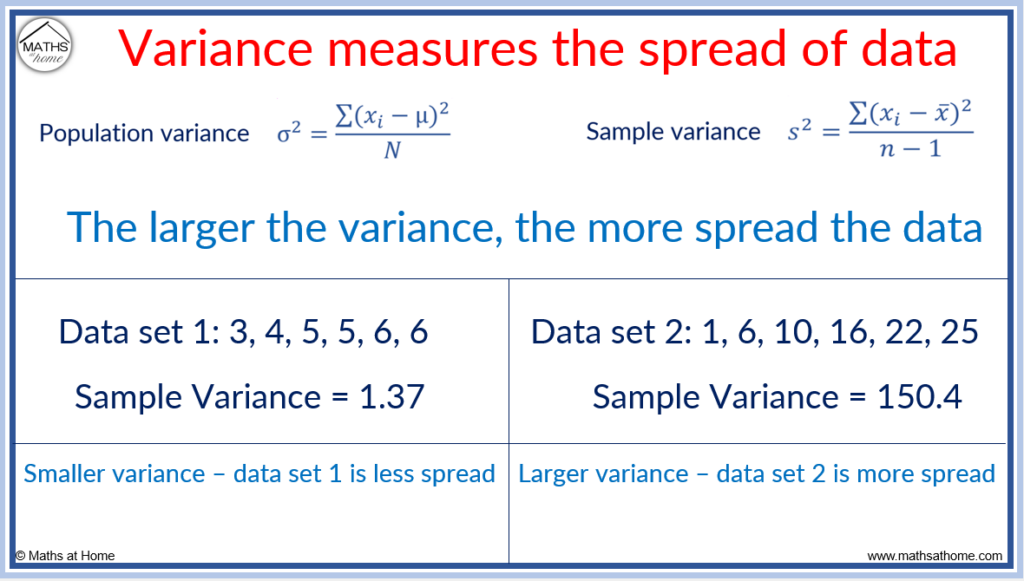
How To Calculate Variance Mathsathome Variance measures how far a data set is spread out. definition, examples of variance. step by step examples and videos; statistics made simple!. When we want to find how each data point in a given population varies or is spread out, then we use the population variance. it is used to give the squared distance of each data point from the population mean. the formula for population variance is written as, \sigma^2 = \dfrac {\sum (x i – \bar {x})^2 } {n} σ2 = n∑(xi–xˉ)2. where,. Variance is a parameter or a statistic that measures how spread out data is relative to its mean. we calculate variance as the average of the squared deviations from the mean. measures of spread like variance are important in statistics because they give you additional information about your data. Variance is the expected value of the squared variation of a random variable from its mean value, in probability and statistics. informally, variance estimates how far a set of numbers (random) are spread out from their mean value. the value of variance is equal to the square of standard deviation, which is another central tool. Calculating variance is a crucial step in data analysis, as it helps to measure the dispersion of data points from the mean value. variance is a fundamental concept in statistics, and it has numerous applications in various fields, including finance, economics, and social sciences. in this article, we will delve into the world of variance calculation, exploring its significance, types, and. To find the variance, take a data point, subtract the population mean, and square that difference. repeat this process for all data points. then, sum all of those squared values and divide by the number of observations. hence, it’s the average squared difference.

Comments are closed.