The Logistic Equation And Models For Population Example 1 Part 1
Solved Problem 1 The Logistic Equation Models Population Chegg In this video, we have an example where biologists stock a lake with fish and after one year the population has tripled. knowing the carrying capacity, i find a formula for the the. Describe the concept of environmental carrying capacity in the logistic model of population growth. draw a direction field for a logistic equation and interpret the solution curves. solve a logistic equation and interpret the results.

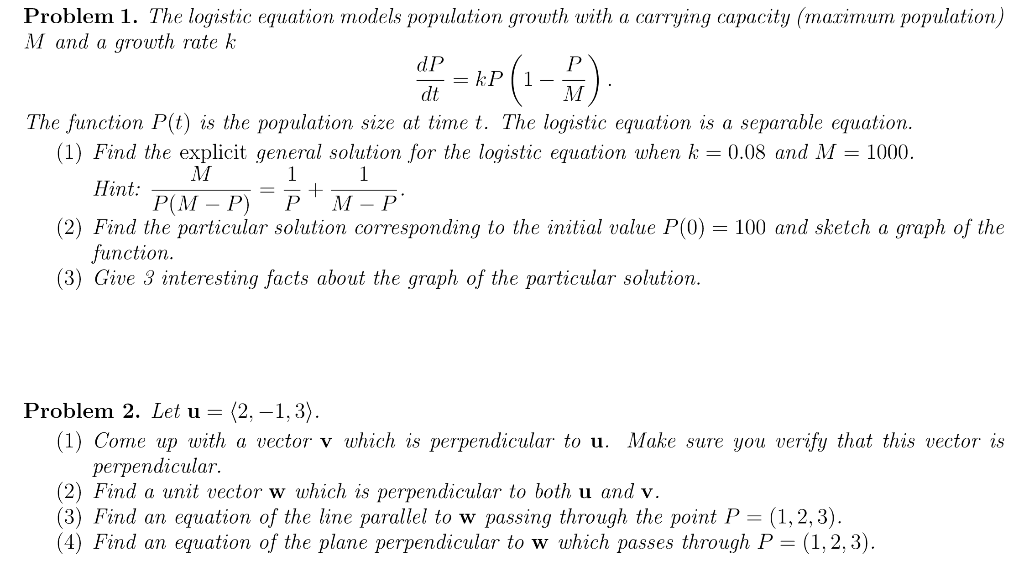
Solved Sources 1 Introduction In This Exercise You Will Chegg Logistic differential equation. in this video we look at the logistic differential equation and its solution. we use the solution to determine when a population will reach a certain size. The equation \(\frac{dp}{dt} = p(0.025 0.002p)\) is an example of the logistic equation, and is the second model for population growth that we will consider. we expect that it will be more realistic, because the per capita growth rate is a decreasing function of the population. The logistic equation and models for population example 1, part 2. in this video, patrick finds how long it actually takes for the population of fish to reach a total of 4,000. show step by step solutions. Example 1: suppose a species of fish in a lake is modeled by a logistic population model with relative growth rate of k = 0.3 per year and carrying capacity of k = 10000. a. write the differential equation describing the logistic population model for this problem. b. determine the equilibrium solutions for this model.
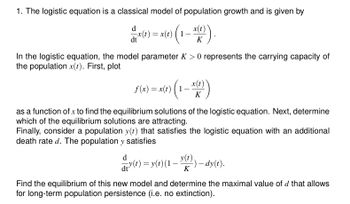
Answered 1 The Logistic Equation Is A Classical Model Of Population The logistic equation and models for population example 1, part 2. in this video, patrick finds how long it actually takes for the population of fish to reach a total of 4,000. show step by step solutions. Example 1: suppose a species of fish in a lake is modeled by a logistic population model with relative growth rate of k = 0.3 per year and carrying capacity of k = 10000. a. write the differential equation describing the logistic population model for this problem. b. determine the equilibrium solutions for this model. Part 1: background: logistic modeling a biological population with plenty of food, space to grow, and no threat from predators, tends to grow at a rate that is proportional to the population that is, in each unit of time, a certain percentage of the individuals produce new individuals. The logistic population model suppose 400 sh are put into a lake with a carrying capacity of 10,000. this means when the population reaches 10,000, it will stop increasing. let y(t) be the population at time t. a di erential equation that models this is the logistic equation: dy dt = ky(10;000 y) = 10;000ky(1 y 10;000). 1.predict its population in 1900 and in 1950 using the exponential model for population growth. 2.in 1900, the population of the us was actually only 76 million people. using this fact, create a logistic model of population growth. 3.use the logistic model from (b) to correct your prediction about the population in 1950. solution:. The logistic equation and models for population example 1, part 1 modeling population with simple differential equation more free lessons at: the logistic.

Solved The Logistic Equation Models The Growth Of A Chegg Part 1: background: logistic modeling a biological population with plenty of food, space to grow, and no threat from predators, tends to grow at a rate that is proportional to the population that is, in each unit of time, a certain percentage of the individuals produce new individuals. The logistic population model suppose 400 sh are put into a lake with a carrying capacity of 10,000. this means when the population reaches 10,000, it will stop increasing. let y(t) be the population at time t. a di erential equation that models this is the logistic equation: dy dt = ky(10;000 y) = 10;000ky(1 y 10;000). 1.predict its population in 1900 and in 1950 using the exponential model for population growth. 2.in 1900, the population of the us was actually only 76 million people. using this fact, create a logistic model of population growth. 3.use the logistic model from (b) to correct your prediction about the population in 1950. solution:. The logistic equation and models for population example 1, part 1 modeling population with simple differential equation more free lessons at: the logistic.

Comments are closed.